Show Mobile NoticeShow All Notes

The AP Calculus AB exam has two sections: AP Calculus AB Exam Past Papers. Section I contains 45 multiple-choice questions for which you are given 105 minutes to complete. AP Calculus AB and BC Course and Exam Description About AP College Board’s Advanced Placement® Program (AP®) enables willing and academically prepared students to pursue college-level studies—with the opportunity to earn college credit, advanced placement, or both—while still in high school. Through AP courses. Here is a set of notes used by Paul Dawkins to teach his Calculus I course at Lamar University. Included are detailed discussions of Limits (Properties, Computing, One-sided, Limits at Infinity, Continuity), Derivatives (Basic Formulas, Product/Quotient/Chain Rules L'Hospitals Rule, Increasing/Decreasing/Concave Up/Concave Down, Related Rates, Optimization) and basic Integrals (Basic Formulas.

Section 2-9 : Continuity

1. The graph of (fleft( x right)) is given below. Based on this graph determine where the function is discontinuous. Solution
2. The graph of (fleft( x right)) is given below. Based on this graph determine where the function is discontinuous. Solution

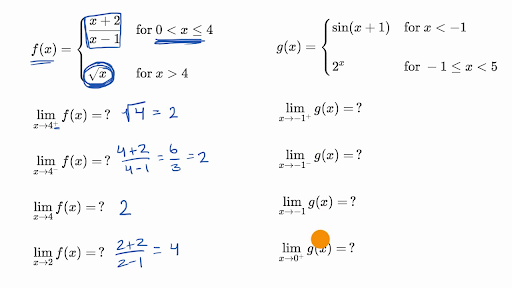
For problems 3 – 7 using only Properties 1 – 9 from the Limit Properties section, one-sided limit properties (if needed) and the definition of continuity determine if the given function is continuous or discontinuous at the indicated points.

1. (displaystyle fleft( x right) = frac{{4x + 5}}{{9 - 3x}})
   1. (x = - 1)
   2. (x = 0)
   3. (x = 3)
   Solution
2. (displaystyle gleft( z right) = frac{6}{{{z^2} - 3z - 10}})
   1. (z = - 2)
   2. (z = 0)
   3. (z = 5)
   Solution
3. (gleft( x right) = left{ {begin{array}{rl}{2x}&{x < 6}{x - 1}&{x ge 6}end{array}} right.)
   1. (x = 4)
   2. (x = 6)
   Solution
4. (hleft( t right) = left{ {begin{array}{rl}{{t^2}}&{t < - 2}{t + 6}&{t ge - 2}end{array}} right.)
   1. (t = - 2)
   2. (t = 10)
   Solution
5. (gleft( x right) = left{ {begin{array}{rc}{1 - 3x}&{x < - 6}7&{x = - 6}{{x^3}}&{ - 6 < x < 1}1&{x = 1}{2 - x}&{x > 1}end{array}} right.)
   1. (x = - 6)
   2. (x = 1)
   Solution

For problems 8 – 12 determine where the given function is discontinuous.

1. (displaystyle fleft( x right) = frac{{{x^2} - 9}}{{3{x^2} + 2x - 8}}) Solution
2. (displaystyle Rleft( t right) = frac{{8t}}{{{t^2} - 9t - 1}}) Solution
3. (displaystyle hleft( z right) = frac{1}{{2 - 4cos left( {3z} right)}}) Solution
4. (displaystyle yleft( x right) = frac{x}{{7 - {{bf{e}}^{2x + 3}}}}) Solution
5. (gleft( x right) = tan left( {2x} right)) Solution

Ap Calculus Ab Problems

For problems 13 – 15 use the Intermediate Value Theorem to show that the given equation has at least one solution in the indicated interval. Note that you are NOT asked to find the solution only show that at least one must exist in the indicated interval.

Calculus Ab & Bcmath Problem Solving Problems

1. (25 - 8{x^2} - {x^3} = 0) on (left[ { - 2,4} right]) Solution
2. ({w^2} - 4ln left( {5w + 2} right) = 0) on (left[ {0,4} right]) Solution
3. (4t + 10{{bf{e}}^t} - {{bf{e}}^{2t}} = 0) on (left[ {1,3} right]) Solution